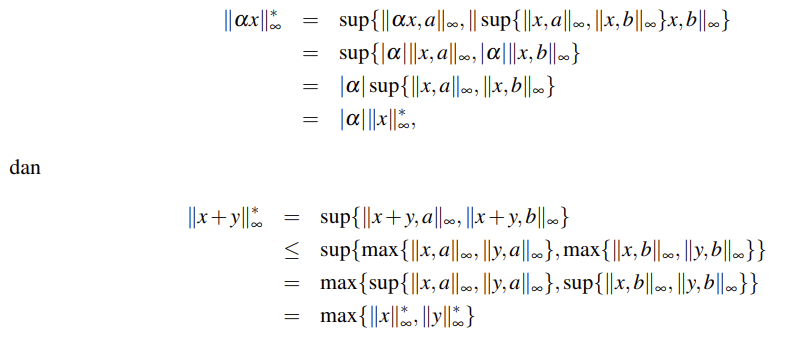Norma-2 non-Archimedean Pada ‘¥(K)
Article Sidebar
Published:
Dec 28, 2021
Keywords:
:lapangan bernilai non-Archimedean, Norma non-Archimedean, Norma-2 non-Archimedean, Ruang Barisan,
Main Article Content
Abstract
Diberikan K merupakan lapangan bernilai non-Archimedean. Pada paper ini dikonstruksikan
norma-2 non-Archimedean pada ruang barisan ‘¥(K). Selanjutnya, dikaji mengenai hubungan
antara norma-2 non-Archimedean dan norma non-Archimedean pada ruang tersebut, sehingga diperoleh hubungan antara kelengkapan ruang ‘¥(K) terhadap norma-2 non-Archimedean dan norma non-Archimedean.
Downloads
Download data is not yet available.
Article Details
How to Cite
Nurnugroho, B. A. (2021). Norma-2 non-Archimedean Pada ‘¥(K). Quadratic: Journal of Innovation and Technology in Mathematics and Mathematics Education, 1(2), 131–137. https://doi.org/10.14421/quadratic.2021.012-08
Section
Articles
References
[1]Sadeghi, M. Amyari and Ghadir, ”Isometrics in Non-Archimedean Strictly Convex,” in NONLINEAR
ANALYSIS AND VARIATIONAL PROBLEMS In Honor of George Isac, T. M. R. a. A. A. K. Panos M.
Pardalos, Ed., New York, Springer, 2010, pp. 13-22.
[2] A. V. Rooij, Non-Archimedean functional analysis, New York: M. Dekker, 1978.
[3] J. Uma, An Analysis of Some Difference Sequence Spaces and Their Duals Over Non-Archimedean
Fields [thesis] . Kattankulathur: Department Of Mathematics Faculty of Science and Humanities Srm
Institute Of Science And Technology, 2018.
[4] J. Choy and S.H. Ku, ”Characterization on 2-Isometries in non-Archimedean 2-normed Spaces,” Journal of The Chungcheong Mathematical Society, vol. 22, no. 1, pp 65-71, 2001.
[5] H. Dutta, ”On sequence spaces with elements in a sequence of real linear,” Applied Mathematics
Letters, vol. 23, no. 9, pp.1109–1113, 2010.
[6] P. Natarajan, Sequence Spaces and Summability over Valued Fields, Boca Raton: CRC Press, Taylor
& Francis Group, 2020.
[7] O. Tug and M. Do ˘ gan, ”Review on Some Sequence Spaces of p-adic Numbers,” Eurasian Journal of ˘
Science & Engineering, vol. 3, no. 1, pp. 231-237, 2017.
[8] A. Malceski, ”‘¥ as n-normed space,” Mat. Bilten, vol. 21, pp. 103-110, 1997.
[9] H. Gunawan, ”The space of p-summable sequences and its natural n-norm,” Bulletin of the Australian
Mathematical Society, vol. 64, no. 1, pp. 137-147, 2001.
ANALYSIS AND VARIATIONAL PROBLEMS In Honor of George Isac, T. M. R. a. A. A. K. Panos M.
Pardalos, Ed., New York, Springer, 2010, pp. 13-22.
[2] A. V. Rooij, Non-Archimedean functional analysis, New York: M. Dekker, 1978.
[3] J. Uma, An Analysis of Some Difference Sequence Spaces and Their Duals Over Non-Archimedean
Fields [thesis] . Kattankulathur: Department Of Mathematics Faculty of Science and Humanities Srm
Institute Of Science And Technology, 2018.
[4] J. Choy and S.H. Ku, ”Characterization on 2-Isometries in non-Archimedean 2-normed Spaces,” Journal of The Chungcheong Mathematical Society, vol. 22, no. 1, pp 65-71, 2001.
[5] H. Dutta, ”On sequence spaces with elements in a sequence of real linear,” Applied Mathematics
Letters, vol. 23, no. 9, pp.1109–1113, 2010.
[6] P. Natarajan, Sequence Spaces and Summability over Valued Fields, Boca Raton: CRC Press, Taylor
& Francis Group, 2020.
[7] O. Tug and M. Do ˘ gan, ”Review on Some Sequence Spaces of p-adic Numbers,” Eurasian Journal of ˘
Science & Engineering, vol. 3, no. 1, pp. 231-237, 2017.
[8] A. Malceski, ”‘¥ as n-normed space,” Mat. Bilten, vol. 21, pp. 103-110, 1997.
[9] H. Gunawan, ”The space of p-summable sequences and its natural n-norm,” Bulletin of the Australian
Mathematical Society, vol. 64, no. 1, pp. 137-147, 2001.